Introduction
The term evapotranspiration (ET) is commonly used to describe two processes of water loss from land surface to atmosphere, evaporation and transpiration. Evaporation is the process where liquid water is converted to water vapor (vaporization) and removed from sources such as the soil surface, wet vegetation, pavement, water bodies, etc. Transpiration consists of the vaporization of liquid water within a plant and subsequent loss of water as vapor through leaf stomata.
Evaporation and transpiration occur simultaneously and both processes depend on solar radiation, air temperature, relative humidity (i.e. vapor pressure deficit) and wind speed. Transpiration rate is also influenced by crop characteristics, environmental aspects and cultivation practices. Different kinds of plants may have different transpiration rates. Not only the type of crop, but also the crop development, environment and management should be considered when assessing transpiration. For example, when the crop is small, water is predominately lost by soil evaporation because little of the soil surface is covered by the plant, but once the crop is well developed and completely covers the soil, transpiration becomes the main process (Allen et al., 1998).
Reference evapotranspiration (ETo) is defined as the rate at which readily available soil water is vaporized from specified vegetated surfaces (Jensen et al., 1990). Then, reference evapotranspiration is defined as the ET rate from a uniform surface of dense, actively growing vegetation having specified height and surface resistance, not short of soil water, and representing and expanse of at least 100 m of the same or similar vegetations (Allen et al., 2005). The concept of the ETo was introduced to study the evaporative demand of the atmosphere independent of crop type, crop development and management practices. If water is abundantly available at the reference surface, soil factors do not affect ET, however, ET may decrease overtime as soil water content decreases. Relating ET to a specific surface provides a reference to which ET from other surfaces can be related. It obviates the need to define a separate ET level for each crop and stage of growth and is referred to as crop ET (ETc). ETo values measured or calculated at different locations or in different seasons are comparable as they refer to the ET from the same reference surface. The only factors affecting ETo are climatic parameters and ETc can be determined from ETo using a crop specific coefficient (Kc).
The use of Kc with specific crops is the subject of other EDIS documents: Basic Irrigation Scheduling in Florida (AE111; Smajstrla et al., 1997), Outline for Managing Irrigation of Florida Citrus with High Salinity Water (AE217; Boman and Stover, 2002), A Web-Based Irrigation Scheduling Model to Improve Water Use Efficiency and Reduce Nutrient leaching for Florida Citrus (SS499; Morgan et al., 2009), Irrigation Scheduling for Tropical Fruit Groves in South Florida (AE21; Migliaccio and Li, 2000), Smart Irrigation controllers: Programming Guidelines for Evapotranspiration-Based Irrigation Controllers (AE445; Dukes et al., 2009) and Principle and Practices of Irrigation Management for Vegetables (Simonne et al., 2010).
A large number of empirical methods have been developed over the last 50 years to estimate evapotranspiration from different climatic variables. Some of these derived from the now well-known Penman equation (Penman, 1948) to determine evaporation from open water, bare soil and grass (now called evapotranspiration) based on a combination of an energy balance and an aerodynamic formula, given as equation [1]:
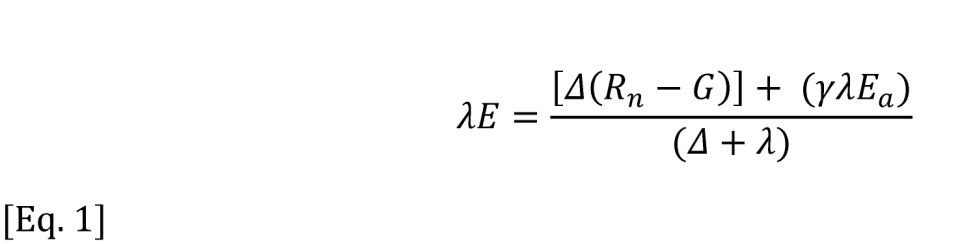
where λE= evaporative latent heat flux (MJ m-2 d-1), Δ = slope of the saturated vapor pressure curve [δeo/ dT, where eo = saturated vapor pressure (kPa) and Tmean = daily mean temperature (oC)]; Rn = net radiation flux (MJ m-2 d-1), G = sensible heat flux into the soil (MJ m-2 d-1), ? = psychrometric constant (kPa oC-1), and Ea = vapor transport of flux (mm d-1).
Various derivation of the Penman equation included a bulk surface resistance term (Monteith, 1965) and the resulting equation is now called the Penman-Monteith equation, which may be expressed for daily values as equation [2]:
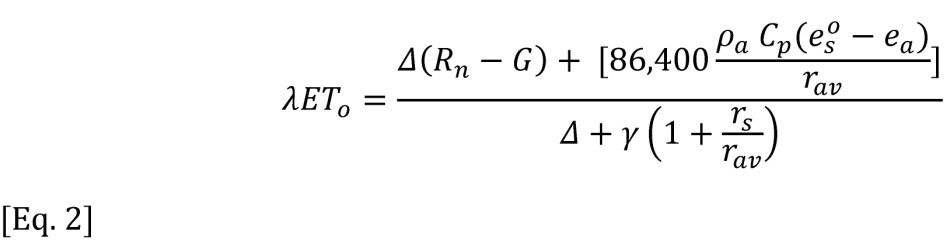
where ?a = air density (kg m-3), Cp = specific heat of dry air, eso = mean saturated vapor pressure (kPa) computed as the mean eo at the daily minimum and maximum air temperature (oC), rav = bulk surface aerodynamic resistance for water vapor (s m-1), ea = mean daily ambient vapor pressure (kPa) and rs = the canopy surface resistance (s m-1).
An updated equation was recommended by FAO (Allen et al. 1998) with the FAO-56 Penman-Monteith Equation, simplifying equation [2] by utilizing some assumed constant parameters for a clipped grass reference crop. It was assumed that the definition for the reference crop was a hypothetical reference crop with crop height of 0.12 m, a fixed surface resistance of 70 s m-1 and an albedo value (i.e. portion of light reflected by the leaf surface) of 0.23 (Smith et al., 1992). The new equation is given as equation [3]:
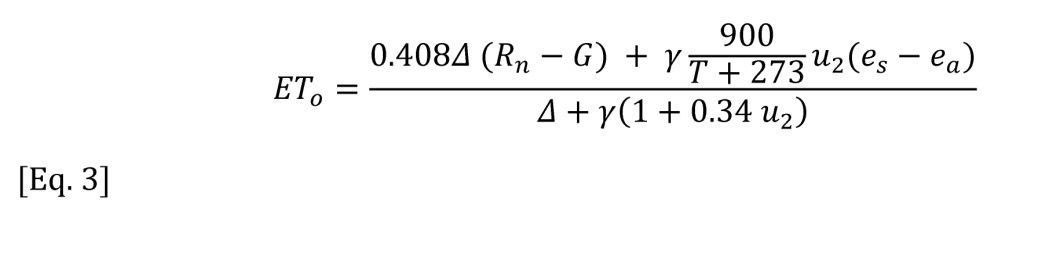
where ETo = reference evapotranspiration rate (mm d-1), T = mean air temperature (oC), and u2 = wind speed (m s-1) at 2 m above the ground. Equation 3 can be applied using hourly data if the constant value “900” is divided by 24 for the hours in a day and the Rn and G terms are expressed as MJ m-2 h-1.
In 1999, the Irrigation Association (IA) requested the Evapotranspiration in Irrigation and Hydrology Committee – Environmental and Water Resources Institute (American Society of Civil Engineering)(ASCE-ET) to establish one standardized equation for estimating the parameters to gain consistency and wider acceptance of ET models (Howell and Evett, 2006). The principal outcome was that two equations, one for a short crop (similar to clipped, cool-season grass) named ETos and another for a tall crop (similar to full-cover alfalfa) named ETrs, were developed for daily (24 hr) and hourly time periods. The ASCE-EWRI standardized reference ET equation based on the FAO 56 Penman-Monteith equation (4) for a hypothetical crop is given as equation [4]:
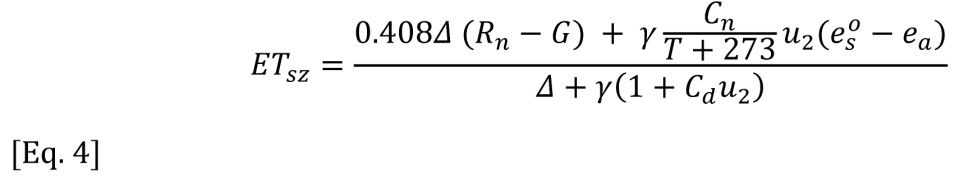
where ETsz = the standardized reference evapotranspiration for grass (ETos) or alfalfa (ETrs) in units based on the time step of mm d-1 for a 24-h day or mm h-1 for an hourly time step, Cn = the numerator constant for the reference crop type and time step and Cd = the denominator constant for the reference crop type and time step (see Table 1 for values of Cn and Cd).
Note that ETsz for a short crop (ETos) equation is identical to the FAO-56 Penman-Monteith equation previously described [Eq. 3]. FAO 56 equation has been selected because it closely approximates grass ETo at the location evaluated, is physically based and explicitly incorporates both physiological and aerodynamic parameters. FAO-56 Penman-Monteith equation has been widely use for Florida conditions. Moreover, procedures have been developed for estimating missing climatic parameters (Allen et al., 2005; Romero et al., 2009).
The objective of this publication is to provide a step-by-step calculation of the reference evapotranspiration (FAO-56 method) for a given location from the available weather data.
Table 1. Values for Cn and Cd in Eq. 4 (after Allen et al., 2005).
Required parameters to calculate ETo
Reference evapotranspiration estimation method is based on climatic data, which can be obtained from a local weather station or, for Florida can be obtained accessing the Florida Automated Weather Network (FAWN, http://fawn.ifas.ufl.edu/). The equation uses standard climatological records of solar radiation (sunshine), air temperature, humidity and wind speed. To ensure the integrity of computations, the weather measurements should be made at 2 m (or converted to that height) above an extensive surface of green grass, shading the ground and not short of water. Table 2 shows a list of parameters required to calculate ETo.
Table 2. Required inputs for ETo calculation.
Overall equation of Penman-Monteith
The FAO Penman-Monteith method to estimate ETo can be derived [Eq. 3]:
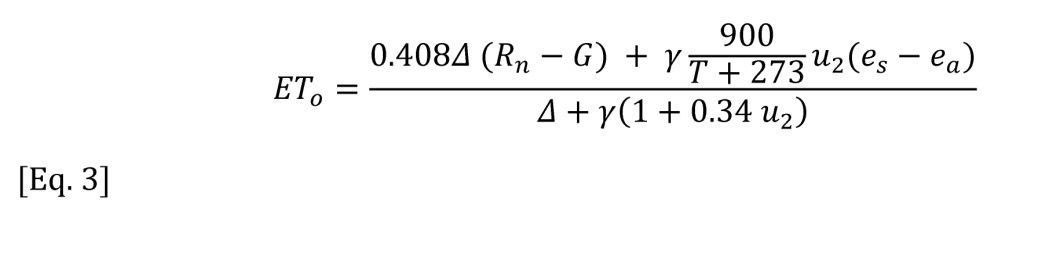
where,
ETo = reference evapotranspiration, mm day-1;
Rn = net radiation at the crop surface, MJ m-2 d-1;
G =soil heat flux density, MJ m-2 d-1;
T =mean daily air temperature at 2 m height, ºC;
u2 =wind speed at 2 m height, m s-1;
es =saturation vapor pressure, kPa;
ea =actual vapor pressure, kPa;
es-ea = saturation vapor pressure deficit, kPa;
? =slope of the vapor pressure curve, kPa ºC-1;
? = psychrometric constant, kPa ºC-1.
The reference evapotranspiration, ETo, provides a standard to which:
- evapotranspiration at different periods of the year or in other regions can be compared;
- evapotranspiration of other crops can be related.
ETo - Practical calculation steps
Step 1 – Mean daily temperature
The (average) daily maximum and minimum air temperatures in degrees Celsius (°C) are required. Where only (average) mean daily temperatures are available, the calculations can still be executed but some underestimation of ETo will probably occur due to the non-linearity of the saturation vapor pressure - temperature relationship (Allen et al., 1998). Average temperature is calculated by [Eq. 5]:

where,
Tmean = mean daily air temperature, ºC;
Tmax = maximum daily air temperature, ºC;
Tmin = minimum daily air temperature, ºC.
Step 2 – Mean daily solar radiation (Rs)
The average daily net radiation expressed in megajoules per square meter per day (MJ m-2 day-1) is required. A simple average of solar radiation values obtained from a weather station in the period of 24h (0:00:01 am to 11:59:59 pm) is required. The conversion of units may be required when solar radiation is expressed in watts per square meter per day (W m-2 day-1). The conversion is given as equation [Eq.6]:
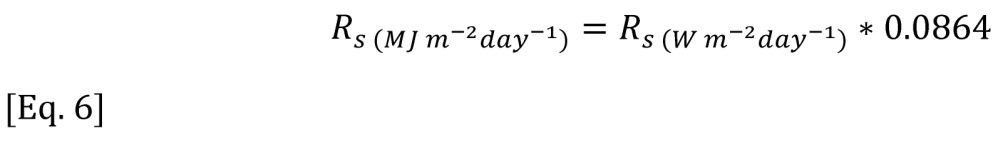
Step 3 – Wind speed (u2)
The average daily wind speed in meters per second (m s-1) measured at 2 m above the ground level is required. It is important to verify the height at which wind speed is measured, as wind speeds measured at different heights above the soil surface differ. The wind speed measured at heights other than 2 m can be adjusted according to the follow equation [7]:
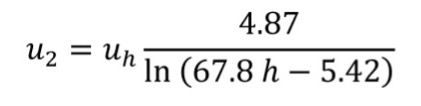
where,
u2 = wind speed 2 m above the ground surface, m s-1;
uz = measured wind speed z m above the ground surface, m s-1;
h = height of the measurement above the ground surface, m.
In case of wind speed is given in miles per hour (mi h-1) the conversion to m s-1 is given as equation [Eq.8]:
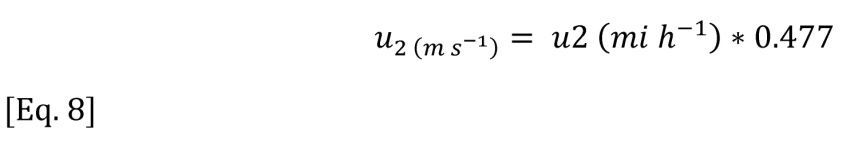
Step 4 - Slope of saturation vapor pressure curve (?)
For the calculation of evapotranspiration, the slope of the relationship between saturation vapor pressure and temperature, ?, is given as equation [Eq.9]:
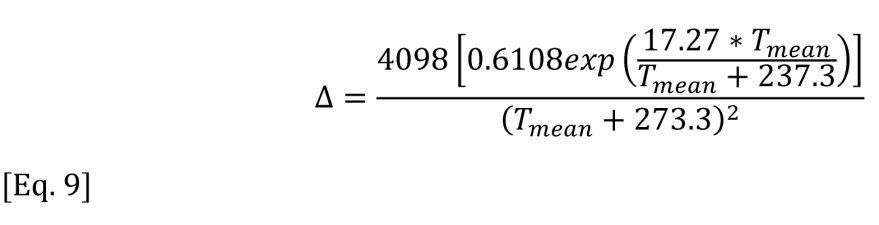
Tmean = mean daily air temperature, ºC, [Eq.5];
exp = 2.7183 (base of natural logarithm).
Step 5 – Atmospheric Pressure (P)
The atmospheric pressure, P, is the pressure exerted by the weight of the earth's atmosphere. Evaporation at high altitudes is promoted due to low atmospheric pressure. This effect is, however, small and in the calculation procedures, the average value for a location is sufficient. A simplification of the ideal gas law, assuming 20°C for a standard atmosphere, can be employed to calculate P in kPa at a particular elevation [Eq. 10]:
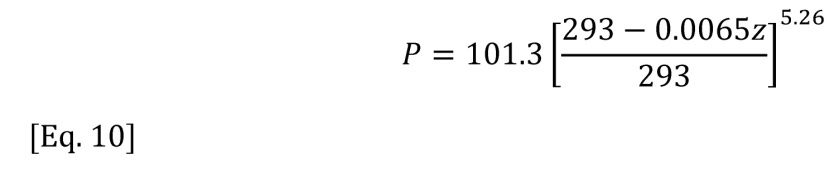
where,
z = elevation above sea level, m.
P = atmospheric pressure, kPa.
Step 6 – Psychrometric constant (?)
The psychrometric constant relates the partial pressure of water in air to the air temperature so that vapor pressure can be estimated using paired dry and wet thermometer bulb temperature readings. Another way to describe the psychrometric constant is the ratio of specific heat of moist air at constant pressure (Cp) to latent heat of vaporization. The specific heat at constant pressure is the amount of energy required to increase the temperature of a unit mass of air by one degree at constant pressure. Its value depends on the composition of the air, i.e., on its humidity. For average atmospheric conditions a cp value of 1.013 10-3 MJ kg-1 °C-1 can be used. As an average atmospheric pressure is used for each location, the psychrometric constant is kept constant for each location depending of the altitude [see Eq. 10]. Psychrometric constant is given as [Eq. 11]:
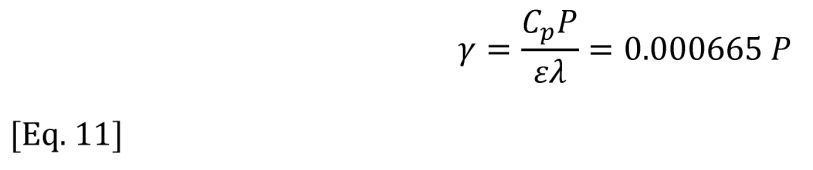
= psychrometric constant, kPa °C-1;
P = atmospheric pressure, kPa, [Eq. 10];
= latent heat of vaporization, 2.45, MJ kg-1;
cp = specific heat at constant pressure, 1.013 10-3, MJ kg-1 °C-1;
= ratio molecular weight of water vapour/dry air = 0.622.
Step 7 – Delta Term (DT) (auxiliary calculation for Radiation Term)
In order to simplify the ETo calculation, several terms are calculated separated. The delta term is used to calculate the “Radiation Term” of the overall ETo equation [see Eq. 33]. DT term is given as [Eq.12]:
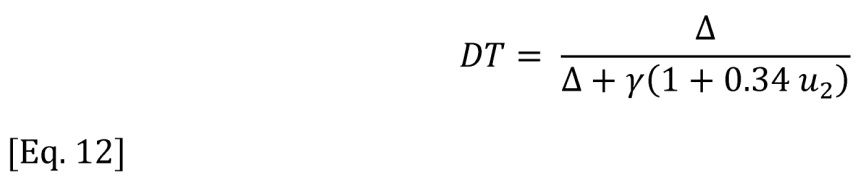
where,
? = slope of saturation vapor curve [Eq.9];
= psychrometric constant, kPa °C-1, [Eq.11];
u2 = wind speed 2 m above the ground surface, m s-1, [Eq.7].
Step 8 – Psi Term (PT) (auxiliary calculation for Wind Term)
The psi term is used to calculate the “Wind Term” of the overall ETo equation [see Eq. 34]. PT term is given as [Eq. 13]:

where,
? = slope of saturation vapor curve [Eq.9];
= psychrometric constant, kPa °C-1, [Eq.11];
u2 = wind speed 2 m above the ground surface, m s-1, [Eq.9].
Step 9 – Temperature Term (TT) (auxiliary calculation for Wind Term)
The temperature term is used to calculate the “Wind Term” of the overall ETo equation [see Eq. 34]. Temperature term is given as [Eq.14]:
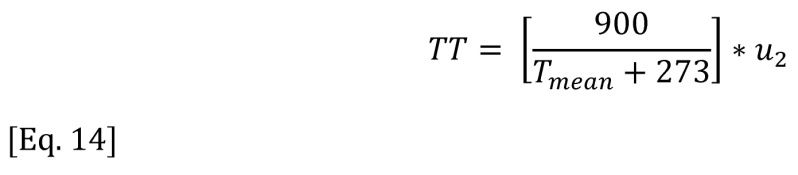
where,
Tmean = mean daily air temperature, ºC, [Eq.5].
Step 10 – Mean saturation vapor pressure derived from air temperature (es)
As saturation vapor pressure is related to air temperature, it can be calculated from the air temperature. The relationship is expressed by [Eq. 15]:
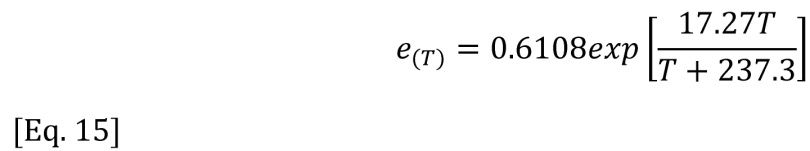
where,
e(T) = saturation vapor pressure at the air temperature T, kPa
T = air temperature, ºC.
Therefore, the mean saturation vapor pressure is calculated as the mean between the saturation vapor pressure at both the daily maximum [Eq. 16]:
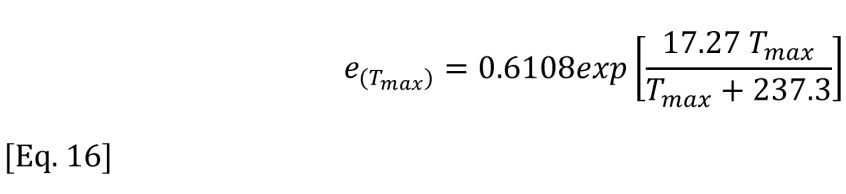
and minimum air temperatures [Eq.17]:
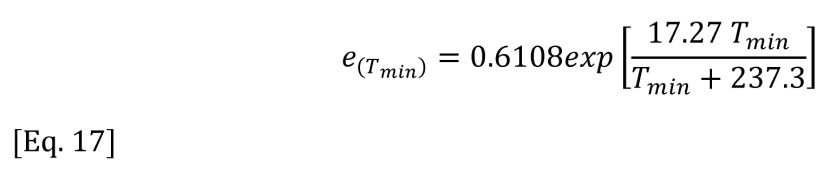
where,
Tmax = maximum daily air temperature, ºC;
Tmin = minimum daily air temperature, ºC.
The mean saturation vapor pressure for a day, week, decade or month should be computed as the mean between the saturation vapor pressure at the mean daily maximum and minimum air temperatures for that period [Eq. 18]:
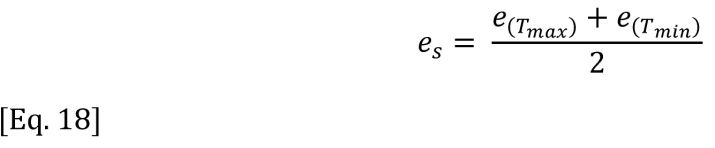
Step 11 – Actual vapor pressure (ea) derived from relative humidity
The actual vapor pressure can also be calculated from the relative humidity. Depending on the availability of the humidity data, different equations should be used [Eq. 19]:
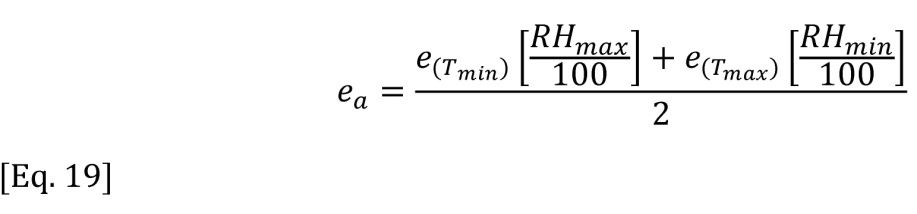
where,
ea = actual vapour pressure, kPa;
e(Tmin) = saturation vapour pressure at daily minimum temperature, kPa, [Eq. 17];
e(Tmax) = saturation vapour pressure at daily maximum temperature, kPa, [Eq. 16];
RHmax = maximum relative humidity, %;
RHmin = minimum relative humidity, %.
Note I: When using equipment where errors in estimating RHmin can be large, or when RH data integrity are in doubt, use only RHmax [Eq. 20]:

In the absence of RHmax and RHmin [Eq.21]:
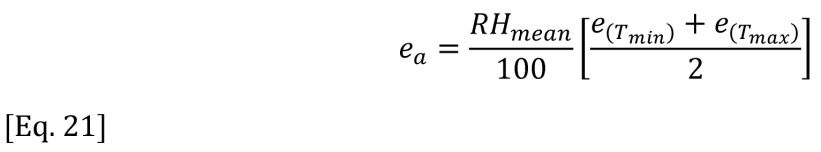
Note II: For missing or questionable quality of humidity data, the ea can be obtained by assuming when the air temperature is close to Tmin, the air is nearly saturated with water vapor and the relative humidity is near 100%, in other words, dewpoint temperature (Tdew) is near the daily minimum temperature (Tmin). If Tmin is used to represent Tdew then [Eq. 22]:
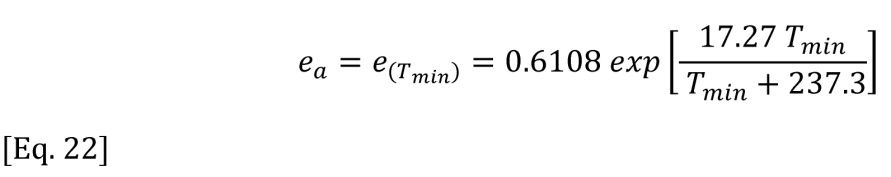
Step 12 – The inverse relative distance Earth-Sun (dr) and solar declination (d)
The inverse relative distance Earth-Sun, dr, and the solar declination, d, are given by equation [Eq. 23]:
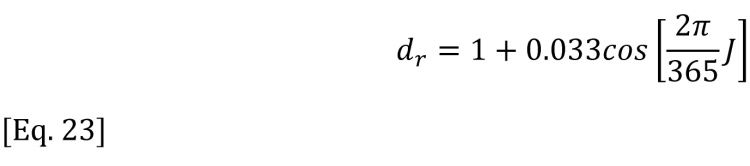
and [Eq. 24]:
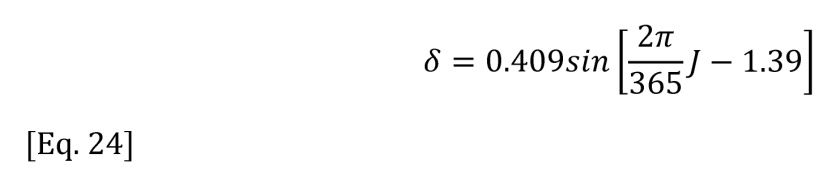
where,
J = number of the day in the year between 1 (1 January) and 365 or 366 (31 December).
Note: to convert date (MM/DD/YYYY) to Julian in Microsoft Excel the following command can be used:
= ((MM/DD/YYYY)-DATE(YEAR((MM/DD/YYYY)), "01","01")+1)
Step 13 – Conversion of latitude (f) in degrees to radians
The latitude, f, expressed in radians is positive for the northern hemisphere and negative for the southern hemisphere (see example below). The conversion from decimal degrees to radians is given by [Eq. 25]:
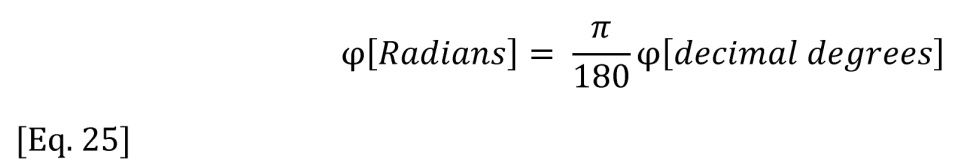
e.g.1. to convert 13º44’N to decimal degrees = 13+44/60 = 13.73
e.g.2. to convert 22º54’S to decimal degrees = (-22)+(-54/60) = -22.90
Step 14 - Sunset hour angle (?s)
The sunset hour angle (?s) is given by [Eq. 26]:
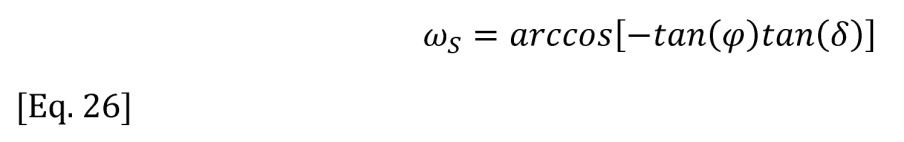
where,
f = latitude expressed in radians, [Eq. 25];
d = solar declination, [Eq. 24];
Step 15 – Extraterrestrial radiation (Ra)
The extraterrestrial radiation, Ra, for each day of the year and for different latitudes can be estimated from the solar constant, the solar declination and the time of the year by [Eq. 27]:
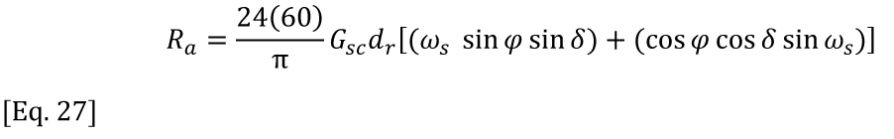
where,
Ra = extraterrestrial radiation, MJ m-2 day-1;
Gsc = solar constant = 0.0820 MJ m-2 min-1;
dr = inverse relative distance Earth-Sun [Eq.23];
?s = sunset hour angle, rad, [Eq. 26];
f = latitude, rad, [Eq.25];
? = solar declination, rad, [Eq. 24].
Step 16 – Clear sky solar radiation (Rso)
The calculation of the clear-sky radiation is given by [Eq. 28]:
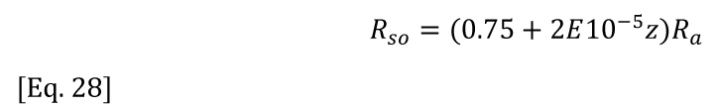
where,
z = elevation above sea level, m;
Ra = extraterrestrial radiation, MJ m-2 day-1, [Eq.27];
Step 17 – Net solar or net shortwave radiation (Rns)
The net shortwave radiation resulting from the balance between incoming and reflected solar radiation is given by [Eq. 29]:

where,
Rns = net solar or shortwave radiation, MJ m-2 day-1;
a = albedo or canopy reflection coefficient, which is 0.23 for the hypothetical grass reference crop, dimensionless;
Rs = the incoming solar radiation, MJ m-2 day-1, [Step 2, Eq.6];
Step 18 – Net outgoing long wave solar radiation (Rnl)
The rate of longwave energy emission is proportional to the absolute temperature of the surface raised to the fourth power. This relation is expressed quantitatively by the Stefan-Boltzmann law. The net energy flux leaving the earth's surface is, however, less than that emitted and given by the Stefan-Boltzmann law due to the absorption and downward radiation from the sky. Water vapor, clouds, carbon dioxide and dust are absorbers and emitters of longwave radiation. It is thereby assumed that the concentrations of the other absorbers are constant. The net outgoing longwave radiation is given by [Eq. 30]:
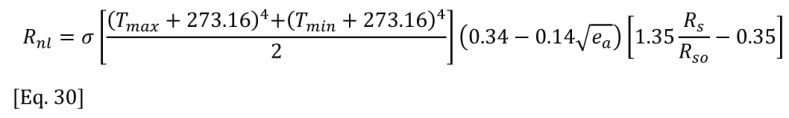
where,
Rnl = net outgoing longwave radiation, MJ m-2 day-1,
s = Stefan-Boltzmann constant [4.903 10-9 MJ K-4 m-2 day-1],
Tmax = K maximum absolute temperature during the 24-hour period [K = °C + 273.16],
Tmin = K minimum absolute temperature during the 24-hour period [K = °C + 273.16],
ea = actual vapor pressure, kPa,
Rs = the incoming solar radiation, MJ m-2 day-1, [Step 2, Eq.6];
Rso = clear sky solar radiation, MJ m-2 day-1, [Step 16, Eq.28];
Step 19 – Net radiation (Rn)
The net radiation (Rn) is the difference between the incoming net shortwave radiation (Rns) and the outgoing net longwave radiation (Rnl), [Eq. 31]:

where,
Rns = net solar or shortwave radiation, MJ m-2 day-1, [Step 17, Eq. 29];
Rnl = net outgoing longwave radiation, MJ m-2 day-1,[Step 18, Eq. 30].
To express the net radiation (Rn) in equivalent of evaporation (mm) (Rng), [Eq. 32]:

where,
Rn = net radiation, MJ m-2 day-1, [Eq. 31];
Final Step – Overall ETo equation
FS1. Radiation term (ETrad)
Radiaton term is given by [Eq. 33]:

where,
ETrad = radiation term, mm d-1;
DT = Delta term, [Step 7, Eq. 12];
Rng = net radiation, mm, [Eq. 32]
FS2. Wind term (ETwind)
Wind term is given by [Eq. 34]:
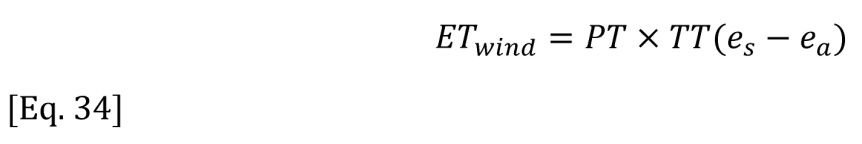
where,
ETwind = wind term, mm d-1;
PT = Psi term, [Step 8, Eq. 13];
TT = Temperature term, [Step 9, Eq. 14];
ea = actual vapor pressure, kPa, [Step 11, Eq. 19];
es = mean saturation vapor pressure derived from air temperature, kPa, [Step 10, Eq. 15];
Final Reference Evapotranspiration Value (ETo)
The final reference evapotranspiration value is the sum of wind and radiation terms, ETo is given by [Eq. 35]:

where,
ETo = reference evapotranspiration, mm d-1;
ETwind = wind term, mm d-1;
ETrad = radiation term, mm d-1;
Summary
The step by step process of reference evapotranspiration calculation was given in this document. Values of evapotranspiration are largely determined by climatic conditions which are available in the FAWN system (http://fawn.ifas.ufl.edu/). The Penman-Monteith ET estimation detailed has been shown to be the most accurate for Florida conditions, and it can be applied on a daily basis for better irrigation management or other water resources applications.
Useful Conversions
1 mm=0.003937 in
1 mm d-1 = 2.45 MJ m-2 d-1
1 J cm-2 d-1 =0.01 MJ m-2 d-1
1 calorie =4.1868 J
1 cal cm-2 d-1 =4.1868 * 10-2 MJ m-2 d-11 W =1 J s-1
1 W m-2 =0.0864 MJ m-2
ºC = (ºF –32) 5/9
Kelvin (ºK) = (ºC) + 273.16
1 millibar (mbar) = 0.1 kPa
1 bar = 100 kPa
1 cm of water = 0.09807 kPa
1 mm of mercury (mmHg) =0.1333 kPa
1 atmosphere (atm) =101.325 kPa
1 lb/in-2 (psi) =6.896 kPa
1 kilometer day-1 (km d-1) =0.01157 m s-1
1 ft s-1 =0.3048 m s-1
References
Allen, R. G., L. S.Pereira, D. Raes, and M. Smith. 1998. "Crop evapotranspiration: guidelines for computing crop water requirements." Irrigation and Drainage Paper No. 56, Food and Agriculture Organization of the United Nations, Rome, Italy.
Allen, R. G., Walter, I. A., Elliot, R. L., Howell, T. A., Itenfisu, D., Jensen, M. E. and Snyder, R. 2005. The ASCE standardized reference evapotranspiration equation. ASCE and American Society of Civil Engineers. https://doi.org/10.1061/9780784408056
ASCE-EWRI 2005. "The ASCE standardized reference evapotranspiration equation." In: Allen RG, Walter IA, Elliott RL, Howell TA, Itenfisu D, Jensen ME, Snyder RL (eds). American Society of Civil Engineers, 69 p.
Blaney, H. F. and Criddle, W. D. 1950. Determining water requirements in irrigated areas from climatological and irrigation data. United States Department of Agriculture, Soil Conservation Service.
Boman, Brian J., and Eddie W. Stover. 2002. “Outline for Managing Irrigation of Florida Citrus With High Salinity Water: ABE332/AE217, 9/2002”. EDIS 2002 (5). Gainesville, FL. https://doi.org/10.32473/edis-ae217-2002.
Dukes, Michael D., Mary L. Shedd, and Stacia L. Davis. 2009. “Smart Irrigation Controllers: Programming Guidelines for Evapotranspiration-Based Irrigation Controllers: AE445/AE445, 2/2009”. EDIS 2009 (2). Gainesville, FL. https://doi.org/10.32473/edis-ae445-2009.
Howell, T. A. and Evett, S. R. 2006. The Penman-Monteith Method. Available online: http://www.cprl.ars.usda.gov/wmru/pdfs/PM%20COLO%20Bar%202004%20corrected%209apr04.pdf. Accessed December 1st, 2009.
Irmak, Suat, and Dorota Z. Haman. 2003. “Evapotranspiration: Potential or Reference? ABE 343/AE256, 6/2003”. EDIS 2003 (14). Gainesville, FL. https://doi.org/10.32473/edis-ae256-2003.
Jensen, M. E., Burman, R. D. and Allen, R. G. 1990. Evapotranspiration and Irrigation Water Requirements. ASCE Manuals and Reports on Engineering Practice No. 70. American Society of Civil Engineers, New York.
McCloud, D. E. 1955. "Water requirements of field crops in Florida as influenced by climate." Proceedings Soil Science Society of Florida 15:165-172.
McCloud, D. E. and Dunavin Jr., L. S. 1954. "The measurement of potential evapotranspiration." The Johns Hopkins University Laboratory of Climatology. Publication in Climatology 7: 55-68.
Migliaccio, Kati W., and Yuncong Li. 2009. “Irrigation Scheduling for Tropical Fruit Groves in South Florida: TR001/TR001, Rev. 1/2009”. EDIS 2009 (2). Gainesville, FL. https://doi.org/10.32473/edis-tr001-2009.
Monteith, J. L. 1965. "Evaporation and Environment." In: The state and movement of water in living organism. 19th Symp. Soc. Exptl. Biol. P. 205-234.
Morgan, Kelly T., Edward A. Hanlon, and Thomas A. Obreza. 2009. “A Web-Based Irrigation Scheduling Model to Improve Water Use Efficiency and Reduce Nutrient Leaching for Florida Citrus: SL286/SS499, 5/2009”. EDIS 2009 (4). Gainesville, FL. https://doi.org/10.32473/edis-ss499-2009.
Penman, H. L. 1948. "Natural evaporation from open water, bare soil and grass." Proceedings of the Royal Society of London. Series A, Mathematical and Physical Sciences, Vol. 193, No. 1032, p. 120-145. https://doi.org/10.1098/rspa.1948.0037
Romero, C. C., Dukes, M. D., Baigorria, G. A., and Cohen, R. 2009. "Comparing theoretical irrigation requirement and actual irrigation for citrus in Florida." Agricultural Water Management 96:473-483. https://doi.org/10.1016/j.agwat.2008.09.021
Simonne, E. H., M. D. Dukes, and L. Zotarelli. 2010. "Principles and Practices of Irrigation Management for Vegetables," p. 17-26, In: S. M. Olson and E. Simonne. eds. Vegetable Production Handbook for Florida 2010-2011.IFAS, Gainesville, FL.
Smajstrla, A. G., B. J. Boman, D. Z. Haman, F. T. Izuno, D. J. Pitts and F. S. Zaxueta. 1997. Basic Irrigation Scheduling in Florida. AE111. Gainesville, University of Florida Institute of Food and Agricultural Sciences, https://edis.ifas.ufl.edu/pdffiles/ae111 Accessed Dec 8, 2009.
Smith, M., Allen, R. G., Monteith, J. L., Pereira, L. S. Perrier, A. and Pruitt, W. O. 1992. Report on the expert consultation on procedures for revision of FAO guidelines for prediction of crop water requirements. Land and Water Development Division, United Nations Food and Agriculture Service, Rome, Italy.
Tabor, P. 1931. "Standard rainfall." Proceedings American Society for Horticultural Science 594-598.
Thornthwaite, C. W. 1948. "An approach toward a rational classification of climate." Geographical Review 38: 55-94. https://doi.org/10.2307/210739